[Genshin Impact Theory] Genshin Shapes - illuminati presentation of random facts and small thoughts about Teyvat's world structure.
Genshin Wiki - Symbols in Teyvat
Triquetra
In the mathematical field of topology, knot theory is the study of mathematical knots. While inspired by knots which appear in daily life, such as those in shoelaces and rope, a mathematical knot differs in that the ends are joined so it cannot be undone, the simplest knot being a ring (or "unknot"). In mathematical language, a knot is an embedding of a circle in 3-dimensional Euclidean space, {\displaystyle \mathbb {R} ^{3}}\mathbb {R} ^{3} (in topology, a circle isn't bound to the classical geometric concept, but to all of its homeomorphisms). Two mathematical knots are equivalent if one can be transformed into the other via a deformation of {\displaystyle \mathbb {R} ^{3}}\mathbb {R} ^{3} upon itself (known as an ambient isotopy); these transformations correspond to manipulations of a knotted string that do not involve cutting it or passing through itself.
The most interesting thing about knots is how many points you need to cut to untie them. Metaphorically Genshin as a whole is a knot or a bunch os knot to untie during our travel.
Knots can be described in various ways. Using different description methods, there may be more than one description of the same knot. For example, a common method of describing a knot is a planar diagram called a knot diagram, in which any knot can be drawn in many different ways. Therefore, a fundamental problem in knot theory is determining when two descriptions represent the same knot.
We have our two siblings who represent the same knot with different path throught Teyvat. Different in time tho.
Cubes
Hypostases are life forms that reached the highest level of elemental purity.
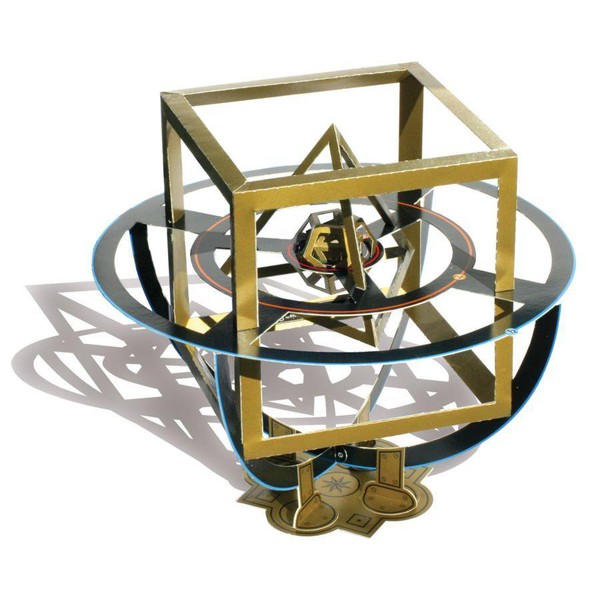
Platonic solid
In three-dimensional space, a Platonic solid is a regular, convex polyhedron. It is constructed by congruent (identical in shape and size), regular (all angles equal and all sides equal), polygonal faces with the same number of faces meeting at each vertex. Five solids meet these criteria:
Cubic honeycomb
A tessellation or tiling of a flat surface is the covering of a plane using one or more geometric shapes, called tiles, with no overlaps and no gaps. In mathematics, tessellations can be generalized to higher dimensions and a variety of geometries.
Tessellations were used by the Sumerians (about 4000 BC) in building wall decorations formed by patterns of clay tiles.
Best example of tiling in Genshin is the visible dome shape of the night sky.
In geometry, the tesseract is the four-dimensional analogue of the cube; the tesseract is to the cube as the cube is to the square.[1] Just as the surface of the cube consists of six square faces, the hypersurface of the tesseract consists of eight cubical cells. The tesseract is one of the six convex regular 4-polytopes.
Why does this all matter do Genshin Imapact?
Theory says that for flatlanders in 2D world we as 3D world creatures would be like gods, who can observe the 2D creatures, even to revert them in space by pulling them to the 3 dimensional worlds. However a 4D creature would be able to do the same with us. It's kind of what the Unkown god does. We can only percept her as 3d creature however her powers come from other dimension(s). Probably the Archon powers too.
More over the Platonic solids are involved into world models, mathematical, that couls as well be the case with Teyvat's world model, which many suggest to be an astrolabe.
More over the Platonic solids are involved into world models, mathematical, that couls as well be the case with Teyvat's world model, which many suggest to be an astrolabe.
This book explains Kepler's cosmological theory, based on the Copernican system, in which the five Platonic solids dictate the structure of the universe and reflect God's plan through geometry. This was the second attempt since Copernicus to say that the theory of heliocentrism is physically true.










Comments
Post a Comment